The recent discovery of colossal magnetoresistance (C M R) effects in the manganese oxide class of materials (such as lanthanum sub 1 minus x, A, sub x, manganese, oxygen sub 3, (where A = strontium, calcium or barium)) has rekindled intense interest in these systems, both because of their fundamental properties as well as their technological potential for read/write heads in magnetic recording media, sensors, and spin-polarized electronics. The role of local charge correlations and their competition with magnetic and electronic ground states is one of the most striking new features of the transition metal oxides such as cuprate superconductors and nickelates, but is most elegantly highlighted for these C M R manganites. Our neutron scattering results near optimal doping show that the short-range polaron correlations are completely dynamic at high T, but then freeze upon cooling to a temperature T sub g. This glass transition suggests that the paramagnetic/insulating state arises from an inherent orbital frustration that inhibits the formation of a long range orbital- and charge-ordered state. Upon further cooling into the ferromagnetic-metallic state, where the polarons melt, the diffuse scattering quickly develops into a propagating, transverse optic phonon.
In the ground state these C M R materials are ideal isotropic ferromagnets. As the system is warmed through the Curie temperature of this half-metallic system the disorder in the spins begins to inhibit the hopping of the (magnetic) conduction electrons, and this in turn provides enough time for the lattice to distort and form polarons that trap the electrons. The ferromagnetic-paramagnetic transition is thus accompanied by a metal-insulator transition; cooling into the ferromagnetic state, or alternatively inducing a magnetization by application of a field, converts the insulator back into a metal, producing the C M R effect.
To investigate the dynamics of these polarons, we have carried out inelastic neutron scattering measurements on a single crystal of optimally-doped lanthanum sub 0.7, calcium sub 0.3, manganese, oxygen sub 3, (T sub C = 252 K) (Refer to reference 1). Figure 1 shows the energy dependence of the polaron scattering at the peak of the polaron correlations (Refer to reference 2). The data reveal that there is a purely elastic component, indicating that the system still possesses order, and since spatially this order is short range in nature we interpret this as a polaron glass.
The data in Figure 1 also exhibit dynamics, in the form of quasielastic scattering (peaking at zero energy). Figure 2 shows the temperature evolution of both the dynamic and purely elastic components, obtained on the optimally-doped C M R bilayer manganite lanthunum sub 1.24, strontium sub 1.76, manganese sub 2, oxygen sub 7 (Refer to reference 3). On decreasing the temperature from 460 K we see that the quasielastic scattering narrows in energy, with a width that decreases approximately linearly until T approximately equal to T star approximately equal to 310 K while the integrated intensity of the scattering is approximately temperature independent. Below approximately 310 K the behavior of the quasielastic width and intensity abruptly changes, and a new, purely elastic component, develops in the spectrum. The elastic scattering develops rapidly below T sub g, and appears to track the resistivity of the material. This increase in the elastic intensity is accompanied by a concomitant decrease of the quasielastic intensity, while the energy width exhibits a change in slope, becoming approximately constant for T sub C < T < T sub g.

| Figure 1. Polaron dynamics at the peak in the correlation function at 260 K, just above T sub C = 252 K, in lanthanum sub 0.7, calcium sub 0.3, manganese, oxygen sub 3. The data reveal a pure elastic signal (indicating an ordered state) and dynamic quasielastic scattering. |
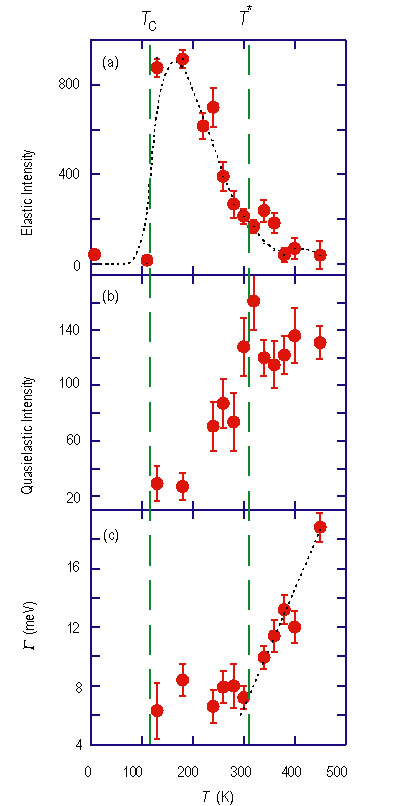
| Figure 2. Temperature dependence of the elastic (A) and quasielastic intensity (B) of the C M R bilayer manganite (T sub C = 114 K). (C) Quasielastic width of the Lorentzian response. Dashed lines are guides to the eye. Error bars correspond to standard deviations obtained from the least squares analysis. |
To further probe the origin of this quasielastic scattering we compiled the map of the dynamical scattering above T star shown in Figure 3. The inelastic response shows that the dynamical scattering still has surprisingly well-defined correlations at these elevated temperatures, demonstrating that the polarons are not isolated Jahn-Teller manganese 3 + deformations, but are dynamically correlated. The dynamical correlation length obtained from these data is approximately 12 angstroms, comparable in size to the static correlation length found at lower T. These dynamic correlations are still present up to 460 K, the highest T explored so far.
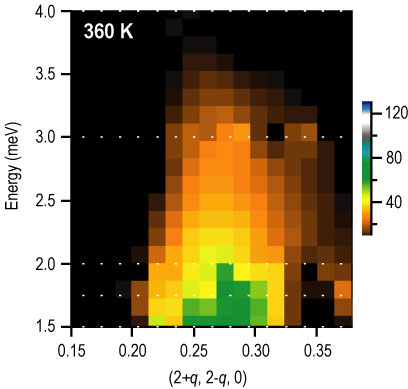
| Figure 3. Dynamic polaron correlations above the glass transition. |
From our measurements we can identify three regimes. For T > T star the observed scattering is dominated by correlations that are completely dynamic. The quasielastic energy width from these correlations varies linearly with T, which is qualitatively what is expected for a continuous phase transition. The second regime occurs for T sub C < T < T star, and is dominated by frozen static polaronic correlations as revealed by the rapid development of the elastic component below T star. The development of the elastic component is accompanied by a decrease of the quasielastic intensity, while the spatial correlations remain short range in nature. This behavior is clear evidence for a freezing transition, analogous to the freezing transition T sub g found in structural glasses or geometrically frustrated spin-glasses. Our data strongly suggest that T star represents a phase transition from a polaronic liquid to a polaronic glass. This polaron glass then melts at T sub C as the ferromagnetic metallic state sets in.
References
[1] J. W. Lynn, F. M. Woodward, D. N. Argyriou, Y. Ren, Y. M. Mukovskii, A. A. Arsenov (preprint).
[2] C. P. Adams, J. W. Lynn, Y. M. Mukovskii, A. A. Arsenov, and D. A. Shulyatev, Phys. Rev. Lett. 85, 3954 (2000); J. Appl. Phys. 89, 6846 (2001).
[3] D. N. Argyriou, J. W. Lynn, R. Osborn, B. Campbell, J. F. Mitchell, U. Ruett, H. N. Bordallo, A. Wildes, and C. D. Ling, Phys. Rev. Lett. 89, 036401 (2002).
Authors
J. W. Lynn and F. M. Woodward
NIST Center for Neutron Research
National Institute of Standards and Technology
Gaithersburg, MD 20899
D. N. Argyriou, R. Osborn, and J. F. Mitchell
Argonne National Laboratory
Argonne, IL 60439
Back to FY2002 HTML main page
Go to next article
To view all symbols correctly, please download Internet Explorer 6 or Netscape 7.1